Systems of Equations No Solution Worksheet
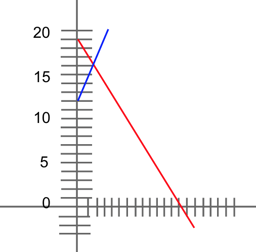
Sure, you've done your paces on single variable equations and now they're no problem, but what do you do when presented with multiple equations and multiple variables at once? These are what we call "systems of equations" and, luckily for us, they are extremely predictable types of problems with multiple methods for solving them. Depending on how you like to work best, you can basically choose your own adventure when it comes to system of equation problems. But before you choose the method that suits you (or the individual problem) best, let's look at all the various options you have available as well as the types of questions you'll see come test day. These questions will always show up once or twice on any given test, so it's best to understand all the strategies you have at your disposal. This will be your complete guide to systems of equations questions—what they are, the many different ways for solving them, and how you'll see them on the SAT. Systems of equations are a set of two (or more) equations which have two (or more) variables. The equations rely on each other and can be solved only with the information that each provides. The majority of the time on the SAT, you will see a system of equations that involves two equations and two variables, but it is certainly not unheard of that you will see three equations and/or a three variables, in any number of combinations. Systems of equations can also be solved in a multitude of ways. As always with the SAT, how you chose to solve your problems mostly depends on how you like to work best as well as the time you have available to dedicate to the problem. The three methods to solve a system of equations problem are: #1: Graphing Let us look at each method and see them in action by using the same system of equations as an example. For the sake of our example, let us say that our given system of equations is: $$2y + 3x = 38$$ $$y - 2x = 12$$ There will only ever beone solution for the system of equations, and that one solution will be the intersection of the two lines. In order to graph our equations, we must first put each equation into slope-intercept form. If you are familiar with lines and slopes, you know that slope intercept-form looks like: $y = mx + b$ So let us put our two equations into slope-intercept form. $2y + 3x = 38$ $2y = -3x + 38$ $y = {-3/2}x + 19$ And $y - 2x = 12$ $y = 2x + 12$ Now let us graph each equation in order to find their point of intersection. Once we graphed our equation, we can see that the intersection is at (2, 16). So our final results are: $x = 2$ and $y = 16$ In order to solve our system of equations through substitution, we must isolate one variable in one of the equations and then use that found variable for the second equation in order to solve for the remaining variable. For example, we have two equations, $2y + 3x = 38$ $y - 2x = 12$ So let us select just one of the equations and then isolate one of the variables. In this case, let us chose the second equation and isolate our $y$ value. $y - 2x = 12$ $y = 2x + 12$ Next, we must plug that found variable into the second equation. (In this case, because we used the second equation to isolate our $y$, we need to plug in that $y$ value into the first equation.) $2y + 3x = 38$ $2(2x + 12) + 3x = 38$ $4x + 24 + 3x = 38$ $24 + 7x = 38$ $7x = 14$ $x = 2$ And finally, you can find the numerical value for your first variable ($y$) by plugging in the numerical value for your second variable ($x$) into either equation. $2y + 3x = 38$ $2y + 3(2) = 38$ $2y + 6 = 38$ $2y = 32$ $y = 16$ Or $y - 2x = 12$ $y - 2(2) = 12$ $y - 4 = 12$ $y = 16$ Either way, you have found the value of both your $x$ and $y$. Again, $x = 2$ and $y = 16$ As the last method for solving systems of equations, you can subtract one of the variables completely in order to find the value of the second variable. We do this by subtracting one of the entire equations from the other, complete, equation. Do take note that you can only do this if the variables in question (the one you wish to eliminate) are exactly the same. If they are NOT the same, then we must first multiply the entire equation by the necessary amount in order to make them the same. In the case of our two equations, none of our variables are equal. $2y + 3x = 38$ $y - 2x = 12$ In this case, let us decide to subtract our $y$ values and cancel them out. This means that we must first make them equal by multiplying our second equation by 2, so that both $y$ values match. $2y + 3x = 38$ $y - 2x = 12$ Becomes: $2y + 3x = 38$ (This first equation remains unchanged) And $2(y - 2x = 12)$ => $2y - 4x = 24$ (The entire equation is multiplied by 2) And now we can cancel out our $y$ values by subtracting the entire second equation from the first. $2y + 3x = 38$ - $2y - 4x = 24$ -------------------- $3x - -4x = 14$ $7x = 14$ $x = 2$ Now that we have isolated our $x$ value, we can plug it into either of our two equations to find our $y$ value. $2y + 3x = 38$ $2y + 3(2) = 38$ $2y + 6 = 38$ $2y = 32$ $y = 16$ Or $y - 2x = 12$ $y - 2(2) = 12$ $y - 4 = 12$ $y = 16$ Our final results are, once again, $x = 2$ and $y = 16$. Though there are many ways to solve your problems, don't let this knowledge overwhelm you; with practice, you'll find the best solving method for you. No matter which method we use to solve our problems, a system of equations will either have one solution—meaning that each variable will have a numerical value attached—no solution, or infinite solutions. In order for a system of equations to have infinite solutions, each system is actually identical. This means that they are the same line. In order for a system of equations to have no solution, the $x$ values will be equal when the $y$ values are set to 1 (which means that both variables—$x$ and $y$—will be equal). The reason this is true is that it will result in two parallel lines, as the lines will have the same slope. The system has no solution because the two lines will never meet and therefore have no point of intersection. For instance, Because our system will have no solution when both our $y$ values and our $x$ values are equal, this means that there will be no solution where we have eliminated both our variables by canceling them out. In this case, the most expedient solution to this problem will be subtraction. Why? We can see this because the two $x$ values ($2x$ and $4x$) are multiples of one another, so we can easily multiply one equation in order to equal them out. $2x - 5y = 8$ $4x + ky = 17$ Now, let us multiply the top equation in order to equal out our $x$ values. So the system pair, $2(2x - 5y = 8)$ $4x + ky = 17$ Becomes, $4x - 10y = 16)$ - $4x + ky = 17$ ---------------------- $-10y - ky = -1$ In order to have NO solution, our two $y$ values must balance out to zero. So let us set our two $y$ values equal to one another: $-10y - ky = 0$ $-ky = 10y$ $k = -10$ Our $k$ valuemust be -10 in order for our system of equations to have no solution. Our final answer is A, -10. [Note: don't fall for the bait answer of +10! You are still subtracting your system of equations, so keep close track of your negatives.] Also, if it is frustrating or confusing to you to try to decide which of the three solving methods "best" fits the particular problem, don't worry about it! You will almost always be able to solve your systems of equations problems no matter which method you choose. For instance, you could have also chosen to graph this question. If you had done so, you would first have to put each equation into slope-intercept form: $2x - 5y = 8$ $4x + ky = 17$ $2x - 5y = 8$ $-5y = -2x + 8$ $y = 2/5(x) + 8$ And $4x + ky = 17$ $ky = -4x + 17$ $y = {-4/k}(x) + 17$ Now, we know a system of equations will have no solution only when each variable balances out to zero, so let us equate our two $x$ variables in order to solve for $k$. $2/5(x) = {-4/k}(x)$ $2/5 = {-4}/k$ ${2k}/5 = -4$ $2k = -20$ $k = -10$ Again, our $k$ value is -10. Our final answer is A, -10. As you can see, there is never any "best" method to solve a system of equations question, only the solving method that appeals to you the most. All roads lead to Rome, so don't stress yourself by trying to find the "right" solving method for your systems problems. Most systems of equations questions on the SAT will let you know that it IS a systems of equations by explicitly using the words "systems of equations" in the question itself. (We will walk through how to solve this question later in the guide) Other problems will simply present you with multiple equations with variables in common and ask you to find the value of a one of the variables, or even a combination of the variables (such as the value of $x + y$ or $x - y$). (We will walk through how to solve this question later in the guide) And finally, the last type of systems of equation question will ask you to find the numerical value of a variable in which there is NO solution, as with the example from earlier. Ready to go beyond just reading about the SAT? Then you'll love the free five-day trial for our SAT Complete Prep program. Designed and written by PrepScholar SAT experts, our SAT program customizes to your skill level in over 40 subskills so that you can focus your studying on what will get you the biggest score gains. Click on the button below to try it out! All systems of equations questions can be solved through the same methods that we outlined above, but there are additional strategies you can use to solve your questions most accurately and expediently. #1: To begin, find the variable that is already the most isolated The ultimate goal is the find the value of all the variables, but we can only do this by finding one variable to start with. The easiest way to solve for this one variable isolate (or eliminate) the variable that has the fewest coefficients or is seemingly the most isolated. For instance, $5x - 3y = -13$ $2x + y = 19$ If we are using substitution, it is easiest for us to first isolate the $y$ value in our second equation. It is already the most isolated variable, as it does not have any coefficients, and so we will not have to deal with fractions once we replace its value in the first equation. If, on the other hand, we were using subtraction, it is still best to target and eliminate our $y$ values. Why? Because we have $3y$ and $y$, which means that we only have to multiply the second equation by 3 in order to match up our $y$ values. If we were to target and eliminate our $x$ values, we would have to multiply both equations—the first by 2 and the second by 5—in order to make our $x$ values match. Though you can always find your solutions no matter which variables you choose to isolate or eliminate, it's always nice to save yourself the time, energy, and hassle (not to mention avoid possible mistakes) by going for the easy pickings first. #2: Practice all three solving methods to see which one is most comfortable to you The best way to decide which system of equation solving method suits you the best is by practicing on multiple problems (though it will help your flexibility if you can become comfortable using all the solving methods available, even if one or two suit you better than the other(s)). When you test yourself on systems questions, try to solve each one using more than one method in order to see which one is most comfortable for you personally. #3: Use subtraction for questions that require finding more than just one variable Most "multiple variable solve" systems of equations questions will ask you to find $x + y$ or $x - y$, which will almost always be most easily found via the subtraction method. It is also most useful to use the method of subtraction when we have three or more variables, especially when it is a combination of multiple variables AND three or more variables. We will see this kind of problem in action in the next section. Now let us test your system of equation knowledge on real SAT math questions. Answers: 300, E, 12 Answer Explanations: 1. As we outlined in our strategy section, it is almost always easiest to find the value of multiple variables by using the method of subtraction (though, again, it is not the only way). We are restricted somewhat, though, as we have three variables and only two equations. Why is this important? Well, we can find the individual values for each variable if we have the same number of equations as we have variables, but in this case we do not. This means we need to use a solution that will give us $x + y$, since we cannot find the value of $x$ or $y$ alone. So let us use subtraction. To do this, we must subtract like variables and, luckily for us, both equations have a single $x + y$ value. This means we can isolate our variable $z$. $x + y + 3z = 600$ $x + y + z = 400$ So let us subtract them. $x + y + 3z = 600$ - $x + y + z = 400$ ------------------------- $2z = 200$ $z = 100$ Now that we have the value of $z$, we can replace it in either of the equations in order to find the value of $x + y$. Because it is always easiest to use the most isolated variable (less math involved for us!), let us our second equation to plug in our $z$ value into. $x + y + z = 400$ $x + y + 100 = 400$ $x + y = 300$ Our final answer for the value of $x + y$ is 300. Do note, however, that if you would much prefer to use substitution, you can definitely do so. Because we are trying to find $x + y$, let us isolate it as our wanted variable in one of our equations. $x + y + 3z = 600$ $x + y + z = 400$ Let us use our first equation. $x + y + 3z = 600$ $x + y = 600 - 3z$ And now we can substitute our $x + y$ value into our second equation. $x + y + z = 400$ $(600 - 3z) + z = 400$ $600 - 2z = 400$ $-2z = -200$ $z = 100$ Now that we have found our value for $z$, we can plug it into either equation to find the numerical value for our $x + y$. Let us use the second equation to do so. Why the second? Because each value is already the most isolated and so will be easiest to work with, but each question will work either way. $x + y + z = 400$ $x + y + 100 = 400$ $x + y = 300$ Again, our final answer is $x + y = 300$ As you can see, any method will suit you—it just depends on how you like to work. 2. Again, though not the only way to solve our problem, it is easiest to use subtraction when we have three or more variables in our equations or we are trying to find a combination of variables (in this case, the value of $y + z$). In this case, we have both, so let us use subtraction. $3x + 2y + 2z = 19$ $3x + y + z = 14$ Our $x$ values are identical, so let us simply subtract the second equation from the first. $3x + 2y + 2z = 19$ - $3x + y + z = 14$ --------------------------- $y + z = 5$ Our final answer is E, $y + z = 5$ 3. In this case, let us use the method of substitution in order to isolate one of our values and plug it into one of the other equations in our system. The equations we are given are: $x = 3v$ $v = 4t$ $x = pt$ $v$ is already isolated, so let us plug it back into our first equation. $v = 4t$ $x = 3v$ $x = 3(4t)$ $x = 12t$ Now, we are also told that $x = pt$, so we can equate the two expressions. $x = 12t$ $x = pt$ $12t = pt$ Because 12 and $p$ both act as coefficients (numbers before a variable) for $t$, we can see that they are equal. This means that $p = 12$ Our final answer is 12. As you can see, systems of equations are some of the most versatile problems when it comes to methods for solving them (though the problems themselves are not terribly varied). Though you can solve many problems on the SAT in a variety of ways, most are not quite so flexible, so take heart that you have many choices for how to proceed for your systems of equations questions. Once you practice and familiarize yourself with these types of questions, you'll find the best method for you—your strengths, and your timing—for taking the test. And pretty soon, you'll be able to knock out systems of equations questions in multiple ways, blindfolded, and with hands behind your back (though why you would want to is, frankly, anyone's guess). Systems were a snap, you say? You're ready for math problems, you say? Well lucky you! We have more math guides than you can shake a stick at, all of which cover crucial aspects of the SAT math section. Lines and angles, polygons, integers, ratios...any topic you need to brush up on is at your fingertips, so make the best of your study time and energy and target any of your known problem areas before test day. Want to know the most valuable strategies for SAT math problems? Check out our guides on plugging in answers and plugging in numbers to help finesse the vast majority of your SAT math questions. Looking to get a perfect score? Look no further than our guide to getting a perfect 800 on the SAT math section, written by a perfect-scorer. Want to improve your SAT score by 160 points? Check out our best-in-class online SAT prep program. We guarantee your money back if you don't improve your SAT score by 160 points or more. Our program is entirely online, and it customizes what you study to your strengths and weaknesses. If you liked this Math strategy guide, you'll love our program. Along with more detailed lessons, you'll get thousands of practice problems organized by individual skills so you learn most effectively. We'll also give you a step-by-step program to follow so you'll never be confused about what to study next. Check out our 5-day free trial: 
What Are Systems of Equations?
#2: Substitution
#3: SubtractionSolving Method 1: Graphing
Solving Method 2: Substitution
Solving Method 3: Subtraction

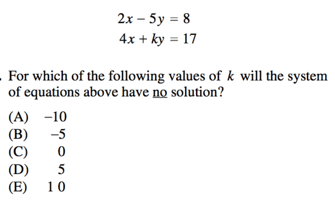

Typical Systems of Equations Questions
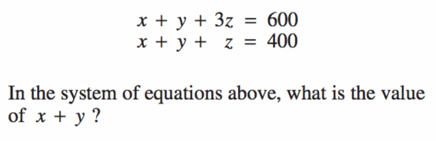
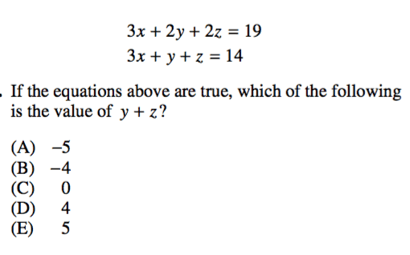
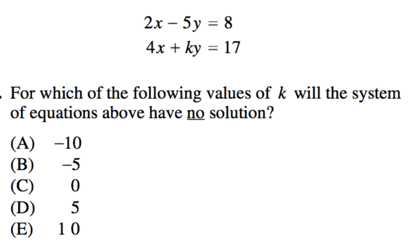

Strategies for Solving Systems of Equations Questions
 Ready to tackle your systems problems and put your strategies to the test?
Ready to tackle your systems problems and put your strategies to the test? Test Your Knowledge
 You did it! Balloons and confetti for you!
You did it! Balloons and confetti for you! The Take-Aways
What's Next?


About the Author
Courtney scored in the 99th percentile on the SAT in high school and went on to graduate from Stanford University with a degree in Cultural and Social Anthropology. She is passionate about bringing education and the tools to succeed to students from all backgrounds and walks of life, as she believes open education is one of the great societal equalizers. She has years of tutoring experience and writes creative works in her free time.
Systems of Equations No Solution Worksheet
Source: https://blog.prepscholar.com/systems-of-equations-sat-math-algebra-prep-and-practice